Consider CHP for LNG Vaporization
The conversion of liquefied natural gas (LNG) to pipeline-quality gas requires large quantities of low-grade thermal energy that may be available from industrial waste streams, steam power plants, or ocean water at the point of discharging LNG from the tanker. Alternatively, heat may be provided by the combustion of LNG or another fuel. In either case, the large temperature differences between these heat sources and the temperature of the LNG can be used to operate an engine that will offset or eliminate the pumping or fuel costs incurred.
North American sources of natural gas continue to decline and, until recently, demand was steadily increasing. The difference between supply and demand was being met by increased imports of liquefied natural gas (LNG).
LNG is created when natural gas (NG) is liquefied by refrigeration at its source. The LNG is transported at near ambient pressure in large insulated tankers across an ocean and then transferred to on-shore or floating off-shore receiving stations with similarly insulated tanks. The liquid, at a temperature of about 110K (–260F), must then be pressurized to pipeline pressure, typically between 3.3 MPa and 10 MPa (500 psi and 1,500 psi), vaporized, and superheated to near ambient temperature before it can be added to an NG transportation or distribution pipeline.
The installation and operating costs of the LNG vaporizing system are major items in the final cost of NG. This article explores some practical and cost-effective combined heat and power (CHP) designs that will improve the energy efficiency of the LNG re-evaporation process.
The composition of NG varies with its source, resulting in slight variations in its thermodynamic properties. That said, NG’s methane content typically is 90% to 95% and, in this article, the analysis is based on the well-known properties of methane alone. The conclusions are qualitatively valid for commercial NG.
The temperature-enthalpy relationship for vaporizing methane is shown in Figure 1. At 3.4 MPa, subcritical methane remains in liquid form up to its boiling point, when it evaporates at constant temperature, and finally heats as a gas to near pipeline temperature. At 10.3 MPa supercritical conditions exist, and the transformation from liquid to gas is continuous with an increase in temperature, and there is no defined boiling point. The difference in the temperature profiles may affect the type of thermodynamic machine chosen for power generation, as discussed later.
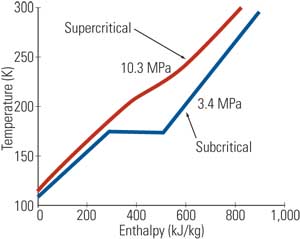
1. Methane goes lower. The relationship between temperature and the heat content of methane is similar to that of water, yet the operating temperature range of methane is much lower. Note that the top line is for a constant pressure of 10.3 MPa (supercritical conditions) and the blue line shows the subcritical change of state at 3.4 MPa. Source: Battelle
Heat Transport via Low-Temperature Organic Rankine Cycle
In the ideal subcritical Rankine cycle, regardless of the working fluid selected, the working fluid is first pressurized and heated as a liquid, evaporated at constant temperature and pressure, superheated at constant pressure, and then expanded isentropically to generate work. The fluid is then condensed at constant temperature and pressure to its original state, and the process repeats.
Figure 2 shows a Rankine cycle (in red) superimposed on representations of the temperature and enthalpy changes of a seawater heat source (a→a’) and a heat sink consisting of a subcritical pressure liquid methane revaporization system (in blue). The physical processes for the subcritical case represented in Figure 2 are detailed in Table 1. Note that 81% of the heat input is conveyed to the methane via the exhaust stream.
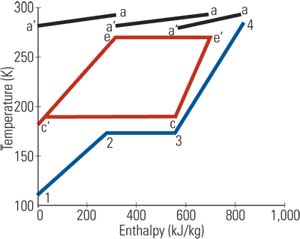
2. Puzzle parts. Temperature-enthalpy plots for an ocean heat source, Rankine power cycle (shown in red) and liquid methane regasification process (shown in green). The working fluid in the Rankine cycle is assumed to be ethane. See Table 1 for data details. Source: Battelle
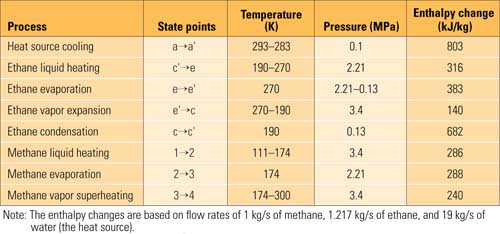
Table 1. Thermodynamic properties for state points shown in Figure 2. Source: Battelle
The conversion of liquefied natural gas (LNG) to pipeline-quality gas requires large quantities of low-grade thermal energy that may be available from industrial waste streams, steam power plants, or ocean water at the point of discharging LNG from the tanker. Alternatively, heat may be provided by the combustion of LNG or another fuel. In either case, the large temperature differences between these heat sources and the temperature of the LNG can be used to operate an engine that will offset or eliminate the pumping or fuel costs incurred.
You may recall from basic thermodynamics class that the classic heat engine requires a heat source and a heat sink at different temperatures in order to produce work. The key to understanding the proposed LNG revaporization processes that follow is that the temperature difference between the heat source (a→a’) and the heat sink supplied by the liquid methane (1→4) is sufficient to operate either a Rankine or a Brayton cycle heat engine. The similarity between the temperature-enthalpy profiles of the Rankine cycle and the methane evaporation process allows the cycle designer to marry those two processes. With the proper choice of the Rankine cycle working fluid, cycle parameters, including the relative mass flow rates of the different working fluids and power production, can be calculated.
In practice, at subcritical methane pressures it is possible to take advantage of the temperature plateaus in the boiling and condensation processes; for supercritical pressures it is probably necessary to use steam extraction between two or more turbine stages to make best use of the heat passing through the system.
Thermodynamic Evaluation
If we assume the adiabatic efficiency of the Rankine cycle ethane turbine is 85%, the conditions listed in Table 1 determine a 20% cycle efficiency at the shaft. Heat rejected in the ethane condenser passes to the liquid methane and supplies 85% of the total heat required for the LNG revaporization. Direct heat transfer supplies most of the balance (above the ethane condensation temperature). Examples of using direct heating to vaporize methane include an air-to-gas heat exchanger and seawater using an open rack heat exchanger, as is used at some existing LNG terminals. Both will eliminate the need for an intermediate (brine) heat transport process. Most seawater-based revaporization plants deliver gas at about 5C (state point 4 in Figure 2).
If the operating conditions defined in Table 2 were applied to a large-scale methane evaporation system rated at 1,000 tonnes per hour, the gross shaft power production rate would be 38.9 MW, or 33 MW net after typical 15% general cycle and parasitic power losses are subtracted. In a typical LNG revaporization system, the imported pumping power required (at least 5 MW for an offshore installation) to bring seawater to a system of open rack heat exchangers is also eliminated. The surplus power would most likely be used on site to offset more expensive purchased power.

Table 2. Thermodynamic properties for state points shown in Figure 4. Source: Battelle
The availability of a waste heat stream for a land-based system at a sustained temperature above about 300K implies that the pumps and piping for the heat source system are already in place. In this case, the heat rejected to the environment is reduced, and all the power generated would be available to offset site power needs. The ethane Rankine power system can be readily integrated with an existing or new steam plant or gas/steam combined-cycle plant that has a once-through cooling system. An evaporative cooling system could be partially or completely replaced by substituting a liquid methane condenser. Calculations show this cycle arrangement could increase the overall output and efficiency of a non-reheat steam plant by 20% to 25% and the output and efficiency of a combined-cycle plant by about 8%.
This proposed power cycle arrangement (Figure 3) may also be applied to a new plant, but it may be wasteful in terms of the cost and parasitic power required for the cooling system. In a custom-designed plant, it would be preferable to condense all the exhaust steam by heat exchange with an independent closed circuit fluid system delivering heat to the liquefied gas evaporator, thereby eliminating much of the conventional cooling water system. The simplest arrangement would be to cool the condenser by direct heat transfer to the ethane circuit, but this could introduce some difficulty in controlling steam condenser pressure because of the large variations in temperature that could occur. Introducing a liquid intermediate heat transport medium could alleviate this problem. The resulting fluid circuits are shown in Figure 4, and the heat balance is given in Table 2.

3. Matching processes. A liquid methane regasification system can use a warm water heat source and a Rankine power generator. Source: Battelle
The overall shaft power efficiency of 38.5% (lines 4 and 14 in Figure 4) assumes the use of a non-reheat steam turbine with a turbine cycle efficiency of about 35%. The ethane turbine increases the overall shaft power by nearly 26% for this case.
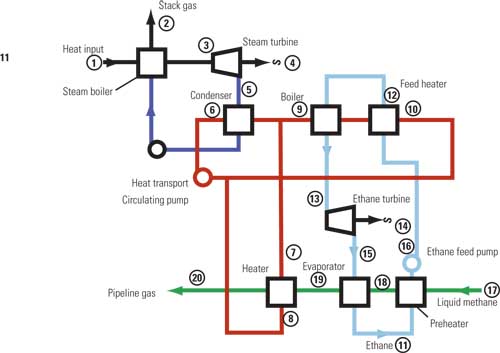
4. Three’s company. A dual Rankine cycle plant with a closed cooling system can efficiently revaporize LNG to pipeline gas. See Table 2 for details on the circled numbers. Source: Battelle
For a given total heat input, a more efficient steam turbine would increase the total power output and the methane evaporation capacity would be decreased. The processing of 1 kg of methane requires 803 kJ of energy, compared to 1,896 kJ supplied per kg of steam, thus enabling the use of more compact heat exchangers than are found in a typical steam plant. The required steam-to-methane ratio is calculated as 803/1,812 = 0.443, and the steam-to-ethane mass flow ratio is 1,547/2,120 = 0.73. A plant evaporating 1,000 tonnes of methane per hour would produce about 180 MW of shaft power, or 160 MW of electrical power, using 1,727 million kJ of gas. Without power production, the gas consumption would be about 900 million kJ, making this a particularly attractive means to not only revaporize LNG but also to generate electrical power. The effective heat rate is very low: (1,727 – 900) / 160,000 = 5,168 kJ/kWh (4,900 Btu/kWh).
Note that the low-temperature organic Rankine cycle LNG evaporation system can also be applied to reciprocating engine cooling systems.
Brayton Cycle Alternative
However, there are practical operational issues that must be considered. Not all plants can match LNG revaporization loads with the power demand when the two cycles are integrated. This situation is exacerbated when electrical power requirements are intermittent yet must rely on the higher thermal inertia of a steam turbine. This limitation, together with the mismatch between the temperature/enthalpy relationships for subcritical steam and supercritical methane systems, may in some cases indicate a need for an alternative to the Rankine power cycle.
The Brayton cycle is powered by a relatively high-temperature heat source: the combustor. However, the closed Brayton cycle with a conventional dry combustion system could supply both power and heat. The resulting unit would have a very high heat utilization factor and would be more environmentally acceptable than the submerged combustion system commonly used in gas-fired evaporation plants.
Figure 5 illustrates an independently fired methane evaporation system incorporating a closed Brayton cycle turbine. The preferred working fluid is an inert, dry gas such as nitrogen. In this configuration the nitrogen circuit is essentially an alternative to the conventional circulating heat transfer medium, such as brine, that would be used in a combustion turbine – based regasification plant.

5. Closed for business. A closed Brayton cycle and liquid methane regasifier appear to be a cost-effective process for gasifying LNG. Source: Battelle
Closed circuit Brayton cycle gas turbines have been studied extensively, but practical difficulties were experienced in the construction of the high-temperature heat exchangers operating at the extremely high turbine inlet temperatures common to today’s advanced technology gas turbines. Also, the low-grade heat rejected by the closed cycle turbine was generally found to be of limited value.
However, with the methane regasifier considered here, the turbine exhaust heat can be fully utilized, and reasonable turbine efficiencies can be achieved with heat source temperatures on the order of 1,000K or lower and moderate turbine inlet temperatures around 800K that are easily managed with modern alloys (Figure 6). In fact, all of the heat supplied to the turbine can be utilized either for power generation or for methane re-evaporation (Table 3).
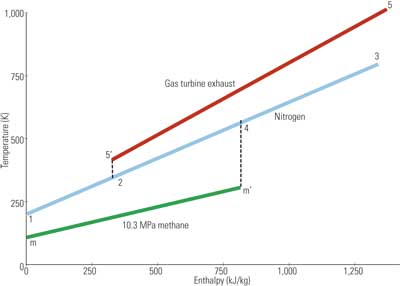
6. Tightly integrated. Temperature-enthalpy plots for a dual Brayton cycle with supercritical methane regasification illustrate how well the cycles integrate thermodynamically. Source: Battelle
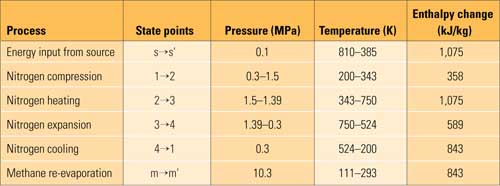
Table 3. Thermodynamic properties forstate points shown in Figure 6. Source: Battelle
Heat is rejected to the methane re-vaporizer between points 4 and 1 in Figure 6. The temperature differentials between the heat supply (s→s’) and the nitrogen and between the nitrogen and the methane can be generous, allowing economical heat exchanger designs and providing tolerance for normal variations in properties of the fluids involved.
You also gain some flexibility in the choice of the physical size of the heat exchangers by selecting nitrogen pressures up to about 0.7 MPa, without risk of nitrogen condensation. The heat given up by 1 kg of nitrogen in cooling from 524K to 200K is 334 kJ, so 843/334 = 2.52 kg of nitrogen are required per kilogram of methane. The values given in Table 3 are based on this nitrogen/methane ratio and also assume an 85% compressor and turbine adiabatic efficiency and internal pressure losses of 7.5% in the nitrogen circuit.
The net power produced by this cycle is proportional to the difference between the enthalpy change during expansion and that during compression, so the cycle efficiency is calculated as (589 – 358) /1,075 = 0.215 or 21.5%. For a methane re-evaporation rate of 1,000 tonnes per hour, the theoretical power developed is 64 MW. After allowing for electrical and gearing losses of 6% and 6 MW for parasitic power (mainly for the flue gas recirculation fan), the electrical power output is calculated as 54 MW, which is comparable to that of a conventional steam power plant, but without the need for external cooling water.
Pumping the large quantities of cooling water required by a conventional utility Rankine power cycle is an expensive proposition. If we assume power costs $50/MWh and the Rankine cycle operates 7,500 hours per year with an evaporation rate of 1,000 tonnes of methane per hour, then the annual power production would be worth $12.375 million. Assuming an incremental investment of $1,000/kW to replace the cooling water system with a methane evaporation system, the payback period would be 2.7 years, assuming that there would be no additional power demand. In reality, the circulation pump capacity would need to be increased by about 17%, so a little over three years would be more realistic. Naturally, all the standard power plant estimating caveats apply to this and the following scoping cost estimates.
Test the Economics
A custom-designed and well-integrated steam/ethane power plant (Figure 4) could produce power at a favorable cost, especially if waste gas streams from boil-off of stored gas or gas treatment units were available. Table 2 shows that the hourly energy demand of a 1,000 tonnes per hour plant at a steam to methane ratio of 0.443 is 1,676,000 MJ. The fuel used that would be required in a typical direct-fired evaporation plant would be 892,000 MJ per hour. The difference, 784,000 MJ, represents the additional fuel used for 160 MW of power generation. With gas at $4.74/GJ ($5/million Btu), this fuel would cost $3,715, or $23.2 per MWh. If the market value of electric power is $50 per MWh, the hourly production is worth $8,000 and the savings are $4,285 per hour.
If the additional plant cost for the integrated regasification system were $2 million per MW of installed capacity, the payback period would be about 10 years, assuming 7,500 hours of operation per year. The plant cost is obviously an estimate, and actual costs depend heavily on the extent to which the generation and re-evaporation operations can be integrated and on the design parameters chosen for the steam cycle, but my calculations are conservative. No doubt the design and costs could be further optimized for a particular installation.
For the independently fired closed Brayton cycle (Figure 5) the only major heat loss is the flue gas — about 14% of the energy input (Figure 7). The marginal energy to be provided by combustion over that required for simple re-evaporation is calculated, as before, as 1,075 – 843 = 232/0.85 = 273 kJ. At a methane evaporation rate of 1,000 tonnes per hour, 273,000 MJ/h of fuel would be required to give a net power output of 54 MW, equivalent to a heat rate of 5.06 kJ per kWh (4,793 Btu per kWh). This corresponds to a fuel cost of 2.4 cents per kWh. The value of power over 7,500 annual hours of operation is $20.25 million against a fuel cost of $9.72 million.
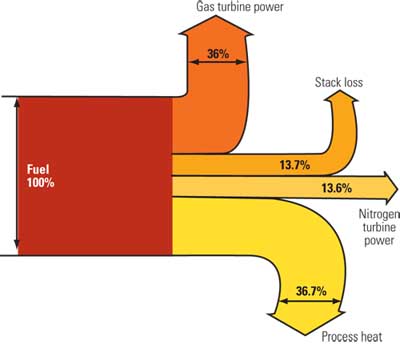
7. Point the way. This Sankey diagram for a dual Brayton cycle regasifier plant shows how the fuel is consumed. The output and efficiency of the dual Brayton turbine system are comparable to those of a conventional gas/steam combined cycle, with no requirement for water or cooling system other than that provided by the methane re-evaporation process. Source: Battelle
For a generating plant costing $1,000 per kW, the simple payback would be a little over five years. The closed Brayton power cycle fuel options are more limited than for the Rankine power cycle, but the moderate heat exchanger temperatures give the Ranking cycle the flexibility to use fuels other than natural gas.
Using these same assumptions, a modern combustion turbine exhausts about 44.6% of its fuel input as waste heat, compared with 36.7% used for the revaporization process using a closed Brayton cycle (Figure 7). For an evaporation rate of 1,000 tonnes per hour at 843 kJ/kg, the energy converted to electric power would be 44.6/36.7 x 843 = 1,024,000 MJ per hour, which is equivalent to 284 MW. The additional hourly fuel cost is $6,186, and the value of the power produced is $14,200. At a cost of $1,000 per kW for the turbo-generator and 7,500 hours of operation per year, the payback period for the Brayton cycle option is also under five years, yet this option uses more than three times the amount of revaporized LNG to run the process.
–Donald Anson ([email protected]) is a research leader at Battelle specializin in energy system design and analysis.